Math Made Easy: Problem of the Day 10

How about getting physical today? Beyond simple addition and multiplication, math was pretty much invented so we could study and predict the physical world. We can use it to describe pretty much every situation we encounter in our environment. So let’s use it to help a cat get a bird.

Our kitty is capable of launching herself at an initial velocity of 5 meters per second. She sees a tasty bird on a branch that is 3 meters up in the air. What is the furthest distance away from the tree the cat can be when she makes her mighty leap, and at what angle does she have to jump? Can our kitty even reach the branch? If not, how low does the branch have to be lowered for her to be able to reach it at all?

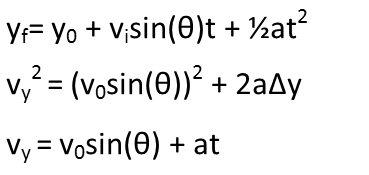
To solve this, we need to look at the equations of projectile motion. The three basic ones we might need, without needing to break our vector into components, look like this for height. The variable v is our velocity with the subscript 0 representing initial velocity and the subscript y the velocity in the y direction at a given time. The variable a is our acceleration in the vertical direction, t our time, theta our angle. For horizontal distance, we just replace the all the y’s with x’s and the sines with cosines.

I actually only need the second one for this problem, so it's the one I'll use. In the vertical direction, the only acceleration we have is that due to gravity, 9.8 m/s², and since it points in the opposite direction of our cat’s motion, it bears a negative sign. As it’s the easiest way to do things, I’ll do my algebra before plugging in any numbers. δy is our height. To find the angle θ at which she needs to jump, I use the height of our branch, and we recognize that, at the apex of her jump, her velocity is zero, since that’s the point where the acceleration due to gravity has cancelled out the momentum of her initial leap. Also note that when I do plug in my numbers, I include my units to make sure the multiply and cancel out correctly – if they do, then I did my algebra right. (When I plugged in my numbers at the end, I just dropped both negative signs since they cancel out).

Oh, my! Look at that! Our poor kitty can’t actually reach the tree. The sine function never returns a number bigger than 1, and here we’re trying to plug 1.53 into the inverse sine. That means kitty isn’t capable of jumping 3 meters straight up. It turns out, if we use the same formula below, where we solve for height and assume the maximum angle is 90°, the highest kitty can reach before gravity starts dragging her back to earth is 1.28m.
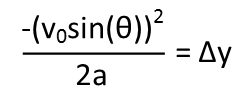
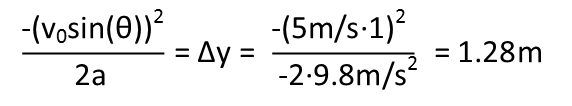