Math Made Easy: Problem of the Day 140

We’ve got some geometry fun for today. As always with any geometry problem, or any physical situation, draw pictures. Start with what you know, and add to your diagram as you think of things that will help solve the problem.

For this one, we can start out be drawing the two circles. Since their centers are a given distance apart, it will be helpful to draw the line connecting their two centers. Then draw the tangent line. It’s a common internal tangent – so that means it crosses in between the two circles – so basically, it runs tangent to the bottom(ish) of one of the two circles and the top(ish) of the other. Also draw the radius of the both circles from their centers to the points of tangency. Make sure to label everything you can.

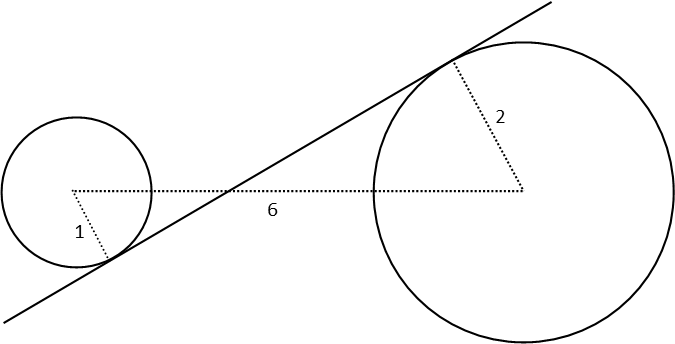
With those basics, we need to think of just how we’re going to find a solution for the problem. Normally, you want to try to find triangles you can draw that are helpful. We already have two triangles, but we don’t really have enough information to solve them. So let’s see if we can find some others. We can, and lots of others, but there’s one that will be most useful, and to see it, we’ll need to draw a figure OTHER than a triangle. Let’s instead make a rectangle. One of the lengths of the rectangle we will have be the tangent line. One of the widths we’ll make be the radius of the small circle. This will actually be very convenient, because it’ll let us know the length of two of its sides. If the rectangle forms the side of a triangle where we know the other two sides, we can find it, and thereby know the tangent line since it is also a length.

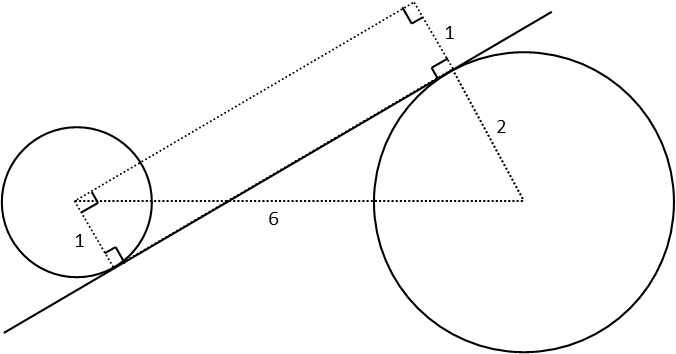
Look at that, we DID form another triangle. And we do know two of its sides – the hypotenuse is the line we drew between the two circles’ centers. One of the bases is the radius of the big circle plus the radius of the small circle. So we can use the Pythagorean Theorem to find the other side, and thereby the tangent line’s length.

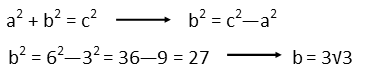
We’ve got our answer. Geometry problems are all about our ability to recognize the relationships of shapes, and find ways to draw solvable shapes to help us find the answers we need. Usually we can do this by forming triangles. But remember – when you can’t find the triangle right off the bat, sometimes drawing another kind of shape you know how to solve will help you find those triangles.