Math Made Easy: Problem of the Day 99

Oft times, when we’re examining physical situations, we ignore certain variables so that we can actually come to a reasonable conclusion. We do this even more so when we’re attempting to teach concepts of mathematics and physics. This is because those variables can overcomplicate the situation and cause it either to be unsolvable, or too complex for the student to grasp. This is one of those situations, and it is called the “three body problem.”

In the three body problem, we have three bodies of relative masses large enough to effect each other through mutual interaction of forces. Here, our acting force is gravity. The three body problem has never been solved. It is considered impossible to solve. The reason being, as each body influences the others, each body moves in accordance to those influences. So the distance between each of the three bodies is constantly changing, which changes how they influence each other. So it becomes an intricate dance that, even with the best models and calculus, is practically impossible to solve. There exist some solution sets to the three body problem, but they all require initial restrictions on the situation.

But don’t worry. This one has a solution, so long as we make certain restrictions and ignore certain variables. In fact, the solution is going to prove ridiculously easy. And we really only need to make three restrictions.

Notice that Bobby’s mass is never mentioned in the problem. This is intentional, and for a couple reasons. First, even a large human’s mass is going to be miniscule compared to masses of celestial objects, even ones as small as the asteroids in our problem (even were she to mass 100kg, she’d only constitute 0.0025% of the mass of the smaller asteroid). I’ll get into the second reason in a moment.

So we’re ignoring Bobby’s mass because it’s insignificant compared to the asteroids. The next assumption we’ll make is that the asteroids are in a fixed position relative to each other – they’re orbiting each other, or something else, stably and in a way that keeps them at a fixed distance from each other, so we don’t have to consider their relative motions.

Thirdly, we’ll assume that Bobby’s velocity relative to the two asteroids is the same and perpendicular to them both. So she’s not starting out already heading more towards one than the other. This allows us to ignore her initial velocity.

Now that we’ve decided we’re going to ignore Bobby’s mass and initial velocity, and restrict the relative motion of the asteroids, we’re ready to consider to which one she’s more strongly attracted. That’ll determine towards which one she drifts. With our restrictions in place, the only force we need to consider is the force of gravity. Thankfully Sir Isaac Newton gave us a handy dandy little equation for this when he formulated his Law of Universal Gravitation back in 1687. It looks a little like this:

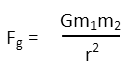
In it, the big G is the universal constant of gravitation – basically a correction factor – m₁ is the mass of one interacting body, m₂ the mass of the other, and r the distance between the center of each interacting body.

To figure out to which one she is more attracted, we simply plug in all our values and compare the results. First we find the force of a gravitic attraction she feels to one asteroid, then the other. But wait! Don’t just jump in and start plugging in numbers now! You can actually save yourself some work. Because we’re comparing the two results, we can actually further ignore things that are the same for both. We simply don’t have to work with those numbers because they’ll cancel. We see the G is the same in both, since that never changes for anything. Ignore it! And Bobby’s mass is the same for both, so not only can we ignore it because it’s statistically insignificant, but we can ignore it because her mass is the same regardless of which of the two asteroids we’re talking about. Fabulous! That means we only have to consider two variables for each value – the mass of each asteroid, and how far that asteroid is from her. Note that we’re also ignoring the size of the asteroid and her body size because those are also insignificant compared to her distance from the asteroids.

So I’m going to set up a comparison, with a question mark in between that we aim to determine whether that question mark is a greater than, equals, or lesser than symbol. With the things we’re able to ignore, it’s going to look like this:

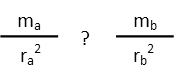
Now we plug in the values for our asteroids.

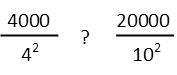
Simplifying, I see that 4 squared has two fours in it, and 4000 has at least one 4 in it. I’m going to cancel a four out of top and bottom there. Likewise, 20000 has at least one 10 in it, as does 10 squared, so I’m going to do the same thing on that side.

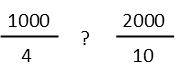
Next, we finish simplifying. 4 goes into 1000 exactly 250 times, and our simplified fraction on the other side is 200. We know that 250 is bigger than 200, so our question mark becomes a greater than symbol.

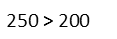
And there we have it. Even though it’s less massive, she’s going to be more strongly attracted to the closer asteroid. That’s because the force of gravity drops off with the square of the distance. So how far away she is matters much more than the mass of the object.

So remember when you’re approaching tough problems that sometimes you can just flat ignore variables that normally affect the situation, but are either insignificant for the one you’re dealing with, or can be fixed to make it less complicated. Figure out what those things are, and do so. That will allow you to find answers where it might seem you’re not able. And for an added challenge, see if you can determine at what point she’ll maintain her distance between the two asteroids.